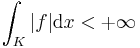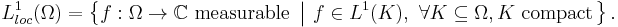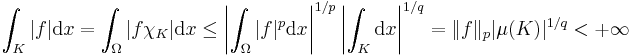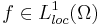Locally integrable function
In mathematics, a locally integrable function is a function which is integrable on any compact set of its domain of definition. Their importance lies on the fact that we do not care about their behavior at infinity.
Contents |
Formal definition
Formally, let  be an open set in the Euclidean space ℝn and
be an open set in the Euclidean space ℝn and 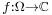 be a Lebesgue measurable function. If the Lebesgue integral of
be a Lebesgue measurable function. If the Lebesgue integral of  is such that
is such that
i.e. it is finite for all compact subsets 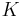 in
in  , then
, then  is called locally integrable. The set of all such functions is denoted by
is called locally integrable. The set of all such functions is denoted by 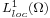 :
:
Properties
Theorem. Every function  belonging to
belonging to 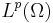 ,
, 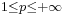 , where
, where  is an open subset of ℝn is locally integrable.
is an open subset of ℝn is locally integrable.
To see this, consider the characteristic function 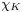 of a compact subset
of a compact subset 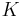 of
of  : then, for
: then, for 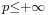
where
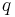 is the positive number such that
is the positive number such that 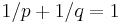 for a given
for a given 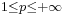
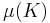 is the Lebesgue measure of the compact set
is the Lebesgue measure of the compact set 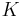
Then by Hölder's inequality, the product 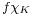 is integrable i.e. belongs to
is integrable i.e. belongs to 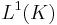 and
and
therefore
Note that since the following inequality is true
the theorem is true also for functions  belonging only to
belonging only to  for each compact subset
for each compact subset 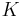 of
of  .
.
Examples
- The constant function
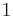 defined on the real line is locally integrable but not globally integrable. More generally, continuous functions and constants are locally integrable.
defined on the real line is locally integrable but not globally integrable. More generally, continuous functions and constants are locally integrable. - The function
- is not locally integrable near
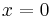 .
.
Applications
Locally integrable functions play a prominent role in distribution theory. Also they occur in the definition of various classes of functions and function spaces, like functions of bounded variation.
See also
References
- Saks, Stanisław (1937), Theory of the Integral, Monografie Matematyczne, 7 (2nd ed.), Warszawa-Lwów: G.E. Stechert & Co., pp. VI+347, JFM 63.0183.05, MR0167578, Zbl 0017.30004, http://matwbn.icm.edu.pl/kstresc.php?tom=7&wyd=10&jez=pl. English translation by Laurence Chisholm Young, with two additional notes by Stefan Banach: the Mathematical Reviews number refers to the Dover Publications 1964 edition, which is basically a reprint.
- Strichartz, Robert S. (2003), A Guide to Distribution Theory and Fourier Transforms (2nd printing ed.), River Edge, NJ: World Scientific Publishers, pp. x+226, ISBN 981-238-430-8, MR2000535, Zbl 1029.46039, http://books.google.it/books?id=T7vEOGGDCh4C&printsec=frontcover&dq=A+Guide+to+Distribution+Theory+and+Fourier+Transforms#v=onepage&q=&f=false.
External links
- Rowland, Todd, "Locally integrable" from MathWorld.
- Vinogradova, I.A. (2001), "Locally integrable function", in Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer, ISBN 978-1556080104, http://www.encyclopediaofmath.org/index.php?title=L/l060460
This article incorporates material from Locally integrable function on PlanetMath, which is licensed under the Creative Commons Attribution/Share-Alike License.